Random element

In probability theory, random element is a generalization of the concept of random variable to more complicated spaces than the simple real line. The concept was introduced by Maurice Fréchet (1948) who commented that the “development of probability theory and expansion of area of its applications have led to necessity to pass from schemes where (random) outcomes of experience can be described by number or a finite set of numbers, to schemes where outcomes of experience represent, for example, vectors, functions, processes, fields, series, transformations, and also sets or collections of sets.”

The modern day usage of “random element” frequently assumes the space of values is a topological vector space, often a Banach or Hilbert space with a specified natural sigma algebra of subsets.

Contents

Definition

Let (Ω, ℱ, P) be a probability space, and (E, ℰ) a measurable space. A random element with values in E is a function X: Ω→E which is (ℱ, ℰ)-measurable. That is, a function X such that for any B ∈ ℰ the preimage of B lies in ℱ: {ω: X(ω) ∈ B} ∈ ℱ.

Sometimes random elements with values in E are called E-valued random variables.

Note if 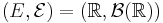(E, \mathcal{E})=(\mathbb{R}, \mathcal{B}(\mathbb{R})), where 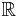\mathbb{R} are the real numbers, and 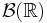\mathcal{B}(\mathbb{R}) is its Borel σ-algebra, then the definition of random element is the classical definition of random variable.

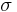The definition of a random element X with values in a Banach space B is typically understood to utilize the smallest \sigma-algebra on B for which every bounded linear functional is measurable. An equivalent definition, in this case, to the above, is that a map 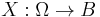X: \Omega \rightarrow B, from a probability space, is a random element if 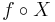f \circ X is a random variable for every bounded linear functional f, or, equivalently, that X is weakly measurable.

Random elements of the various nature

References

  1. ^ a b Stoyan, D., and Stoyan, H. (1994) Fractals, Random Shapes and Point Fields. Methods of Geometrical Statistics. Chichester, New York: John Wiley & Sons. ISBN 0-471-93757-6

Literature

External links